HCF and LCM Questions

May 26, 2022 June 12, 2022

It is for all Competition exams Free mock test (Practice set)/quiz/MCQ is for all types of exams like STET, CTET, SSC, Railway, Bank, SBI and Bank PO, Clerk, Lekhpal, NDA, UPSC and other Competition Exams. You get all types of questions (Quantitative Aptitude) with detailed solutions in Hindi/English on this site.

Donate me through 👇

UPI ID:- achalup41-1@oksbi

1. LCM of two numbers is 225 and HCF is 5. If one number is 25, then the other number will be-||दो संख्याओं का ल. स. 225 है तथा उनका म. स. 5 है। यदि एक संख्या 25 हो, तो दूसरी संख्या होगी-

1. 5

2. 25

3. 45

4. 225

... Answer is 3)
Let the second number||माना दूसरी संख्या = y
LCM✕HCF = 25✕y
225✕5 = 25✕y
45 = y

2. If the LCM and HCF of two expressions are (x²+6x+8)(x+1) and (x+1) respectively and one expression is x²+3x+2 then what will be the other?||यदि दो व्यंजकों का ल. स. और म. स. क्रमशः (x²+6x+8)(x+1) और (x+1) है और एक व्यंजक x²+3x+2 है तो दूसरी क्या होगी?

1. x²+5x+4

2. x²-5x+4

3. x²+4x+5

4. x²-4x+5

... Answer is 1)
Let the second number||माना दूसरी संख्या = y
(x²+6x+8)(x+1)✕(x+1) = (x²+3x+2)✕y
(x+2)(x+4)(x+1)✕(x+1) = (x+2)(x+1)✕y
(x+4)(x+1) = y
x²+x+4x+4 = y
y = x²+5x+4

3. The product of the two numbers is 4107. If HCF is 37, then what will be the largest number among them?||दो संख्याओं का गुणनफल 4107 है। यदि उनका म. स. 37 हो, तो उनमें बड़ी संख्या होगी?

1. 185

2. 111

3. 107

4. 101

... Answer is 2)
Let the two numbers be 37 x and 37 y||माना दो संख्याएँ 37 x और 37 y है
37x✕37y = 4107
xy = ⁴¹⁰⁷⁄_37✕37
xy = 3
possible pair||संभव युग्म = (3, 1)
largest number||बड़ी संख्या = 3✕37 = 111

4. HCF and LCM of two numbers are 44 and 264 respectively. If the first number is divided by 2, then the quotient is 44, which of the following will be the second number?||दो संख्याओं का म. स. और ल. स. क्रमशः 44 और 264 है। यदि पहली संख्या को 2 से भाग दिया जाए, तो भागफल 44 होता है दूसरी संख्या निम्नलिखित में से क्या होगी?

1. 147

2. 528

3. 132

4. 264

... Answer is 3)
First number||पहली संख्या = 2 ✕44 = 88
Let the second number||माना दूसरी संख्या = y
88✕y = 44✕264
y = 132

5. The least number which divided by 4, 6, 8, 12 and 16 leaves a remainder of 2 in each case?||वह छोटी से छोटी संख्या जिसे 4, 6, 8, 12 और 16 से भाग करने पर प्रत्येक दशा में 2 शेष होगा?

1. 46

2. 48

3. 50

4. 56

... Answer is 3)
LCM(4, 6, 8, 12, 16)

Required number||अभीष्ट संख्या = 48+2 =50

6. Find the greatest five digit number which divided by 3, 5, 8, 12 leaves a remainder of 2?||पाँच अंकों की वह सबसे बड़ी संख्या ज्ञात कीजिए जिसे 3, 5, 8, 12 से भाग देने पर 2 शेष बचे?

1. 99999

2. 99958

3. 99960

4. 99962

... Answer is 4)
LCM(3, 5, 8, 12) = 120

Required number||अभीष्ट संख्या = 99999-39+2 =99962

7. The smallest multiple of 13 which divided by 4, 5, 6, 7 and 8 leaves a remainder of 2 in each case is-||13 का वह सबसे छोटा गुणज जिसे 4, 5, 6, 7 और 8 से भाग देने पर प्रत्येक दशा में 2 शेष बचता है-

1. 2520

2. 842

3. 2522

4. 840

... Answer is 3)
LCM(4, 5, 6, 7, 8) = 840
Required number||अभीष्ट संख्या = 840k+2
On putting k =3||k=3 रखने पर,
= 840✕3+2 = 2522 [which is completely divisible by 13|| जो 13 से पूर्णतया विभाजित होगा]

8. Find the greatest four digit number which divided by 15, 18, 21 and 24 leaves remainder 11, 14, 17 and 20 respectively.||चार अंकों की ऐसी सबसे बड़ी संख्या ज्ञात कीजिए जिसे 15, 18, 21 और 24 से भाग देने पर क्रमशः 11, 14, 17 और 20 शेष बचें।

1. 6557

2. 7556

3. 5675

4. 7664

... Answer is 2)
LCM(15, 18, 21, 24) = 2520

(∵ 15-11 = 4, 18-14 = 4, 21-17 = 4, 24-20=4)
Required number||अभीष्ट संख्या = 9999-2439-4 = 7556

9. Find the minimum number of 6 digits which when divided by 4, 6, 10, 15 leaves remainder 2 in each case.||6 अंकों की न्यूनतम संख्या ज्ञात करें जिसे 4, 6, 10, 15 से भाग देने पर प्रत्येक दशा में शेष 2 आता है।

1. 100000

2. 100022

3. 100020

4. 100040

... Answer is 2)
LCM(4, 6, 10, 15) = 60

Minimum number of 6 digits which is divisible by 60||6 अंकों की न्यूनतम संख्या जो 60 से विभाजित हो
∴ 60-40 = 20
= 100000+20 = 100020 (which completely divisible by 60||जो 60 से पूर्णतया विभाज्य है )
Required number||अभीष्ट संख्या = 100020+2 = 100022
[Note:- On subtracting any number in this number, it becomes less than 6 digits, Therefore in the 'minimum number questions' add any number and not subtract.||नोट:- इस संख्या में किसी भी संख्या को घटाने पर यह 6 अंकों से छोटी हो जाती, इसलिए न्यूनतम संख्या के प्रश्नों में जोड़ते है घटते नहीं। ]

10. The smallest number which when divided by 5, 10, 12 and 15 leaves remainder 2 in each case but when divided by 7 leaves no remainder.||वह सबसे छोटी संख्या, जिसे 5, 10, 12 तथा 15 से भाग देने पर प्रत्येक दशा में 2 शेष रहे किन्तु 7 से भाग देने पर कोई शेष न रहे।

1. 189

2. 182

3. 175

4. 91

... Answer is 2)
LCM(5, 10, 12, 15) = 60
∴ Number||संख्या = 60k+2
The required number should be completely divisible by 7.||अभीष्ट संख्या 7 से पूर्णतः विभाज्य होना चाहिए।
= 7✕8k+4k+2
Putting k = 3 in 4k+2 will be divisible by 7.||4k+2 में k = 3 रखने पर 7 से विभाजित होगा ।
Required number||अभीष्ट संख्या = 7✕8✕3 + 4✕3+2
= 168+12+2
= 182

11. What is the smallest number which when divided by 5, 6, 7 and 8 leaves remainder 3 in each case, but when divided by 9 leaves no remainder?||वह सबसे छोटी संख्या कौन सी है जिसमें 5, 6, 7 एवं 8 से भाग देने पर प्रत्येक दशा में 3 शेष रहे, किन्तु 9 से भाग देने पर कुछ शेष न रहे?

1. 1677

2. 1683

3. 2523

4. 3363

... Answer is 2)
LCM(5, 6, 7, 8) = 840
∴ Number||संख्या = 840k+3
The required number should be completely divisible by 9.||अभीष्ट संख्या 9 से पूर्णतः विभाज्य होना चाहिए।
= 9✕93k+3k+3
Putting k = 2 in 3k+3 will be divisible by 9.||3k+3 में k = 2 रखने पर 9 से विभाजित होगा ।
Required number||अभीष्ट संख्या = 9✕93✕2+3✕2+3
= 1674+6+3
= 1683

12. The greatest number which when divided by 1288, 8139, 17200 gives the same remainder.||वह सबसे बड़ी संख्या जो 1288, 8139, 17200 को भाग देने पर समान शेषफल दे।

1. 220

2. 221

3. 300

4. 225

... Answer is 2)
Difference between all numbers||सभी संख्याओं के बीच अंतर
8139-1288 = 6851
17200-8139 = 9061
17200-1288 = 15912

= 13✕17
= 221

13. What is the greatest number which when divided by 411, 684, 821 leaves remainder 3, 4 and 5 respectively?||वह बड़ी से बड़ी संख्या क्या है जिससे 411, 684, 821 को भाग देने पर क्रमशः 3,4 तथा 5 शेष बचता है?

1. 235

2. 356

3. 136

4. 122

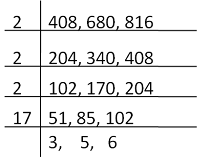
... Answer is 3)
Note:- Remainder is subtracted before calculating HCF||नोट:- HCF निकालने से पहले शेषफल घटाया जाता है
411-3 = 408
684-4 = 680
821-5 = 816
p
= 2✕2✕2✕17
= 136

14. A, B, and C start running at the same time in the same direction from the same point in a circular stadium. 'A' completes a round in 252 seconds, B in 308 seconds and C in 198 seconds. After what time will they meet again?||A, B, C एक ही समय एक वृत्ताकार स्टेडियम में एक ही बिन्दु से एक ही दिशा में भागना शुरू करते है। A एक चक्कर 252 सेकंड में पूरा कर लेता है B 308 सेकण्ड में और C 198 सेकण्ड में। वे कितने समय बाद फिर मिलेंगे ?

1. 26m 18s/26 मिनट 18 सेकण्ड

2. 42m 36s/42 मिनट 36 सेकण्ड

3. 45m/45 मिनट

4. 46m 12s/46 मिनट 12 सेकण्ड

... Answer is 4)
LCM(252, 308, 198)

LCM = 2✕2✕7✕9✕11
= 2772s
they will meet again after 46m 12s||46m 12s बाद फिर मिलेंगे

15. What are the numbers between 100 and 200 which are divisible by both 9 and 6?||100 तथा 200 के बीच आने वाली वे संख्याएँ कितनी है जो 9 तथा 6 दोनों से विभाजित हों?

1. 5

2. 6

3. 7

4. 8

... Answer is 2)
The first number between 100 and 200 that is divisible by 9, 6||100 और 200 के बीच पहली संख्या जो 9, 6 से विभाजित होगी = 108
LCM(9,6) = 18
The number which is divisible by 9 and 6 will also be divisible by 18.||जो संख्या 9 तथा 6 से विभाजित होगी वह 18 से भी विभाजित होगी।

total numbers||कुल संख्याएँ = 6

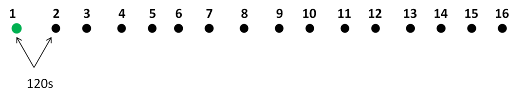
16. Six bells start ringing simultaneously, then at intervals of 2, 4, 6, 8, 10, 12 seconds. How many times will they ring together in 30 minutes?||छः घंटियाँ एक साथ बजना शुरू होती है, फिर 2, 4, 6, 8, 10, 12 सेकण्ड के अंतराल में बजती है। 30 मिनट में वे कितनी बार एक साथ बजेंगी?

1. 4

2. 10

3. 15

4. 16

... Answer is 4)
LCM(2, 4, 6, 8, 10, 12) = 120s
= ^(30✕60)⁄₁₂₀
= 15

We will add 1 more because the bells start ringing together after that it rings every 120s interval.||1 और जोड़ेंगे क्योंकि घण्टियाँ एक साथ बजना प्रारम्भ होती है उसके बाद हर 120s के अंतराल पर बजती है।
=15+1 =16

17. Find HCF of ²⁄_3,⁴⁄₅ and ⁶⁄₇ ||²⁄_3,⁴⁄₅ तथा ⁶⁄₇ का महत्तम समापवर्तक क्या है?

1. ⁴⁸⁄₁₀₅

2. ²⁄₁₀₅

3. ¹⁄₁₀₅

4. ²⁴⁄₁₀₅

... Answer is 2)
HCF of 2/3, 4/5 and 6/7 ||2/3, 4/5 तथा 6/7 का महत्तम समापवर्तक = ^{HCF(2, 4, 6)}⁄_{LCM(3, 5, 7)}
= ²⁄₁₀₅

18. Find LCM of ¹⁄_3,²⁄_9,⁵⁄_6,⁴⁄₂₇ ||¹⁄_3,²⁄_9,⁵⁄_6,⁴⁄₂₇का लघुत्तम समापवर्त्य क्या है?

1. ¹⁄₅₄

2. ¹⁰⁄₂₇

3. ²⁰⁄₃

4. ⁹²⁄₃

... Answer is 3)
LCM of 1/3, 2/9, 5/6, 4/27||1/3, 2/9, 5/6, 4/27 का लघुत्तम समापवर्त्य = ^{LCM(1, 2, 5, 4)}⁄_{HCF(3, 9, 6, 27)}
= ²⁰⁄₃

19. 3^3³³³ +1 और 3^3³³⁴ +1 का महत्तम समापवर्तक कितना है?

1. 2

2. 1

3. 3^3³³⁴ +1

4. 20

... Answer is 1)
3¹ = 3, 3² = 9, 3³ = 27, 3⁴ = 81
∵ In each case, the odd digit is appearing at the unit place.||प्रत्येक स्थिति में इकाई स्थान पर विषम अंक आ रहा है
∴ Adding 1 to these numbers will make them all even numbers, which are divisible by 2.||इन संख्या में 1 जोड़ने पर ये सभी सम संख्या बन जाएंगी, जो 2 से विभाजित होंगी
HCF = 2

20. The product of 2 digit numbers is 2160 and their HCF is 12. What will be both the numbers?||2 अंक की संख्याओं का गुणनफल 2160 है और उनका महत्तम समापवर्तक 12 है। दोनों संख्याएँ क्या होंगी?

1. (12, 60)

2. (72, 30)

3. (36, 60)

4. (60, 72)

... Answer is 3)
HCF = 12
Let the numbers||माना संख्याएँ = 12 x, 12y
12x✕12y = 2160
xy = ²¹⁶⁰⁄_12✕12
xy = 15
= 3✕5 or||या 1✕15
= (3, 5) or (1, 15)
Required numbers||अभीष्ट संख्याएँ = 3✕12 =36, 5✕12 = 60

21. Three numbers are in the ratio 2 : 3 : 4 and their HCF is 12. What is its LCM?||तीन संख्याएँ 2:3:4 के अनुपात में है तथा उनका म. स. 12 है। उनका ल. स. होगा?

1. 144

2. 192

3. 96

4. 72

... Answer is 1)
Let the numbers||माना संख्याएँ = 2x, 3x, 4x
HCF(2x, 3x, 4x) = x = 12
2x = 2✕12= 24
3x = 3✕12 = 36
4x = 4✕12 = 48
LCM(24, 36, 48) = 144
or, short-method
LCM(2x, 3x, 4x) = 12x
HCF = 12
= 12✕12 = 144

22. The ratio of the sum of two numbers with their LCM is 7:12. If their HCF is 4 then what is the smaller number?||दो सँख्याओं के योगफल का उनके ल. स. के साथ अनुपात 7:12 है। यदि उनका म. स. 4 है तो छोटी संख्या है?

1. 20

2. 16

3. 12

4. 8

... Answer is 3)
Let the numbers||माना संख्याएँ = 4x, 4y
LCM(4x, 4y) = 4xy
^(4x+4y)⁄_4xy = ⁷⁄₁₂
12(x+y) = 7xy
If x =3, y =4, then satisfies the above equation||यदि x = 3, y = 4, तो उपरोक्त समीकरण को संतुष्ट करता है
smaller number||छोटी संख्या = 4✕3 = 12

23. The HCF and LCM of two numbers are 21 and 84 respectively. If the ratio of two numbers is 1:4, then the larger of the two numbers will be?||दो संख्याओं का म. स. और ल. स. क्रमशः 21 और 84 है। यदि दो संख्याओं का अनुपात 1:4 है, तो दोनों संख्याओं में से बड़ी संख्या होगी?

1. 12

2. 108

3. 48

4. 84

... Answer is 4)
HCF = 21 Let the numbers||माना संख्याएँ = 21x, 21y
Ratio||अनुपात = 1:4
larger number||बड़ी संख्या = 21✕4 = 84

24. The product of LCM and HCF of two numbers is 24. The difference of both the numbers is 2. Find those numbers?||दो संख्याओं के ल. स. और म. स. का गुणनफल 24 है। दोनों संख्याओं का अंतर 2 है। वे संख्याएँ ज्ञात कीजिए?

1. 8, 6

2. 8, 10

3. 2, 4

4. 6, 4

... Answer is 4)
Let the numbers||माना संख्याएँ = x, y
xy = 24
x-y = 2 ......(i)
∴(x+y)² = (x-y)² + 4xy
(x+y)² = (2)² + 4✕24
(x+y)² = 100
x+y = 10 .....(ii)
From eq. (i)and (ii)
2x = 12
x = 6
y = 4

25. The sum of two numbers is 45 and their HCF and LCM are 3 and 168 respectively, what will be the sum of the reciprocals of those numbers?||दो संख्याओं कस योगफल 45 है तथा उनके म. स. तथा ल. स. कमशः 3 तथा 168 है उन संख्याओं के व्युत्क्रमों का योगफल होगा?

1. ¹⁵⁄₅₇

2. ³⁄₁₁

3. ¹⁵⁄₅₆

4. ⁵⁄₅₆

... Answer is 4)
Let the numbers||माना संख्याएँ = 3x, 3y
3x+3y = 45
x+y = 15 ......(i)
LCM(3x, 3y) = 3xy = 168 .....(ii)
¹⁄_3x + ¹⁄_3y = ^(x+y)⁄_3xy
= ¹⁵⁄₁₆₈
= ⁵⁄₅₆

26. The sum of two numbers is 36 and their HCF is 4. How many pairs of such numbers are possible?||दो संख्याओं का योग 36 है तथा उनका म. स. 4 है। इस प्रकार की संख्याओं के कितने युग्म संभव है?

1. 1

2. 2

3. 3

4. 4

... Answer is 3)
Let the numbers||माना संख्याएँ = 4x, 4y
4x+4y = 36
x+y = 9
pairs of numbers||संख्याओं के युग्म = (1, 8) (2, 7) (4, 5)